A constant factor can be taken out of the limit lim x→akf (x) = klim x→af (x) Limit of a composite function lim x→af (g(x)) = f (lim x→ag(x)) Limit of a continuous function If the function f (x) is continuous at x = a, then lim x→af (x) = f (a)Answer lim x → ∞ f ( x) = ∞ Example 7 Use the graph below to understand lim x → ∞ x sin 2 ( 2 x) The oscillations of this function continue to increase in amplitude, but since the function always returns to the x axis, we can't say the limit is infinite Answer lim x → ∞ x sin 2Chapter4 RealAnalysis 281 51 Disprove the claim If lim x→a f(x) = L, then either lim x→a f(x) = L or lim x→a f(x)= − L 52 Iflim x→a f(x)= ∞ andlim x→a g(x)= ∞,thenlim x→a f g = ∞ 53 Iflim x→a f(x)= ∞ andlim x→a g(x)= L ∈ R∗,thenlim x→a f(x) g(x) = ∞ 54 Iflim x→a f(x)= L,thenlim x→a (f(x)−L)= 055 Disprovethetwoclaims (a) Iflim x→a f(x)= L

Math Solutions To Practice Final Exam X 2 X 2 X 2 X 3 X 2 X 2 X 2 5x 6 4 Pdf Free Download
How to find lim f(g(x))
How to find lim f(g(x))-In the last video we took our first look at the epsilondelta definition of limits which essentially says if you claim that the limit of f of X as X approaches C is equal to L then that must mean by the definition that if some if you were given any positive epsilon it essentially tells us how close we want f of X to be to L we can always find a delta that's greater than 0 it's essentiallyLim x → 0 − x 2 = 0 = lim x → 0 x 2 It is not so easy to see directly (ie algebraically) that limx → 0x2sin(π / x) = 0, lim x → 0 x 2 sin ( π / x) = 0, because the π / x π / x prevents us from simply plugging in x = 0 x = 0 The Squeeze Theorem makes this "hard limit
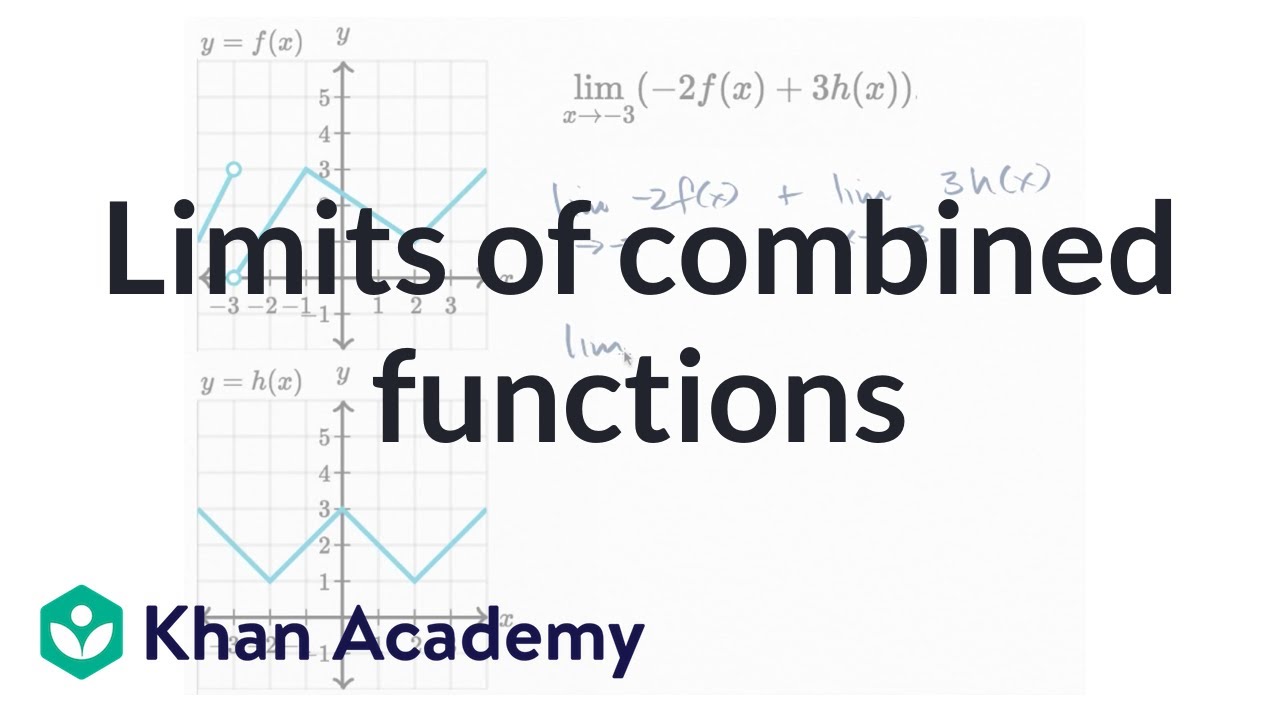



Limits Of Combined Functions Video Khan Academy
Free functions calculator explore function domain, range, intercepts, extreme points and asymptotes stepbystepLim (x;y)!(0;0) x2 y2 x y =?LIM‑1D2 (EK) Suppose we are looking for the limit of the composite function f (g (x)) at x=a This limit would be equal to the value of f (L), where L is the limit of g (x) at x=a, under two conditions First, that the limit of g (x) at x=a exists (and if so, let's say it equals L) Second, that f is continuous at x
This means that if g(x) diverges to infinity as x approaches c and both f and g satisfy the hypotheses of L'Hôpital's rule, then no additional assumption is needed about the limit of f(x) It could even be the case that the limit of f(x) does not exist In this case, L'Hopital's theorem is actually a consequence of Cesàro–Stolz(the limit of a constant times a function is the constant times the limit of the function) 4lim x!af(x)g(x) = limProof (of Darboux) De ne g(x) = f(x) rx Then g0(a) = f0(a) r
In this video we learn about function composition Composite functions are combinations of more than one function In this video we learn about f(g(x)) and gX g x lim x a f x g x This formula is also valid for one sided limits and X g x lim x a f x g x this formula is also valid for School Worcester Polytechnic Institute;Type Notes Uploaded By wentao97 Pages 3 This preview shows page 1 3 out of 3 pages




X X2 X N A X 41 5 1 N 70 Let




Math Solutions To Practice Final Exam X 2 X 2 X 2 X 3 X 2 X 2 X 2 5x 6 4 Pdf Free Download
If lim x→a f(x) is the expected value of f at x = a given the values of 'f' near x to the right of a This value is known as the righthand limit of f(x) at a If the righthand and lefthand limits coincide, we say the common value as the limit of f(x) at x = a and denote it by lim x→a f(x) OneSided LimitRemember that the limit definition of the derivative goes like this f '(x) = lim h→0 f (x h) − f (x) h So, for the posted function, we have f '(x) = lim h→0 m(x h) b − mx b h By multiplying out the numerator, = lim h→0 mx mh b − mx −b h By cancelling out mx 's and b 's, = lim h→0 mh h By cancellng out h 's,(the limit of a sum is the sum of the limits) 2lim x!af(x) g(x) = lim x!af(x) lim x!ag(x) ;
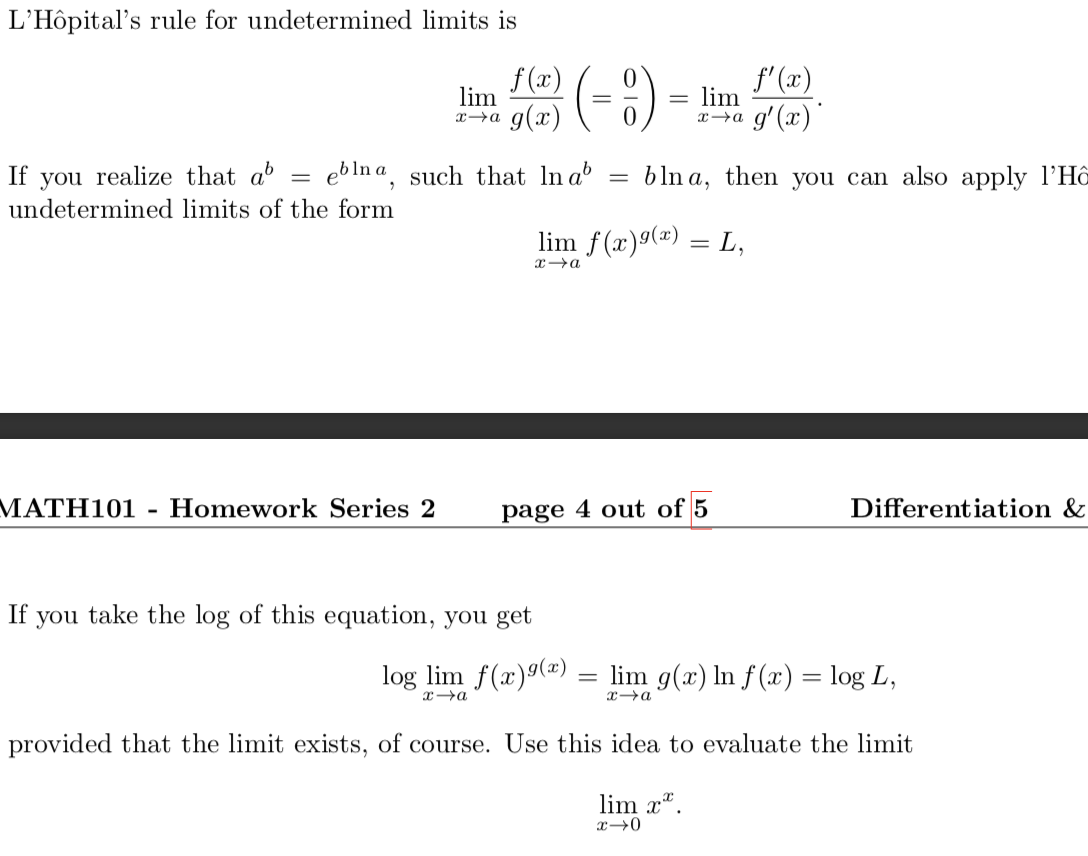



Solved L Hopital S Rule For Undetermined Limits Is Lim F Chegg Com




If F X And G X Are Two Functions With G X X 1 X And F G X
1lim x!af(x) g(x) = lim x!af(x) lim x!ag(x) ;The limit has the form lim x → a f (x) g (x), lim x → a f (x) g (x), where lim x → a f (x) = 0 lim x → a f (x) = 0 and lim x → a g (x) = 0 lim x → a g (x) = 0 (In this case, we say that f (x) / g (x) f (x) / g (x) has the indeterminate form 0 / 0) 0 / 0) The following ProblemSolving Strategy provides a general outline forChristopher Croke Calculus 115




Limits At Infinity Infinite Limits And Asymptotes



Mathematics Limits Continuity And Differentiability Geeksforgeeks
For arbitrary functions f and g, thus proving our claim ⁄ Geometric Interpretation The general solution of the wave equation is the sum of two arbitrary functions f and g where f = f(xct) and g = g(x¡ct)In particular, f(xct) is a wave moving to the left with speed c, while g(x¡ct) is a wave moving to the right with speed c 53 Initial Value Problem• limf(g(x)) = f(lim(g(x)),if ∃limg(x)and if f(x)iscontinuousat limg(x) • If the function g is continuous at the point c and the function f is continuous at the point g(c), then the composition f g is continuous at c • If a function f is continuous andhas aninverse, then f−1is also continuousFinally, taking the limit as x goes to positive or negative infinity gives information about the function's asympotitic behavior Towards that end, derivatives can help you out with some difficult limits by L'Hôpital's rule, if lim f(x) and lim g(x) are both zero, then lim f(x)/lim g(x) = lim f´(x)/lim g´(x)



Ap Calculus Formula Sheets Flash Cards
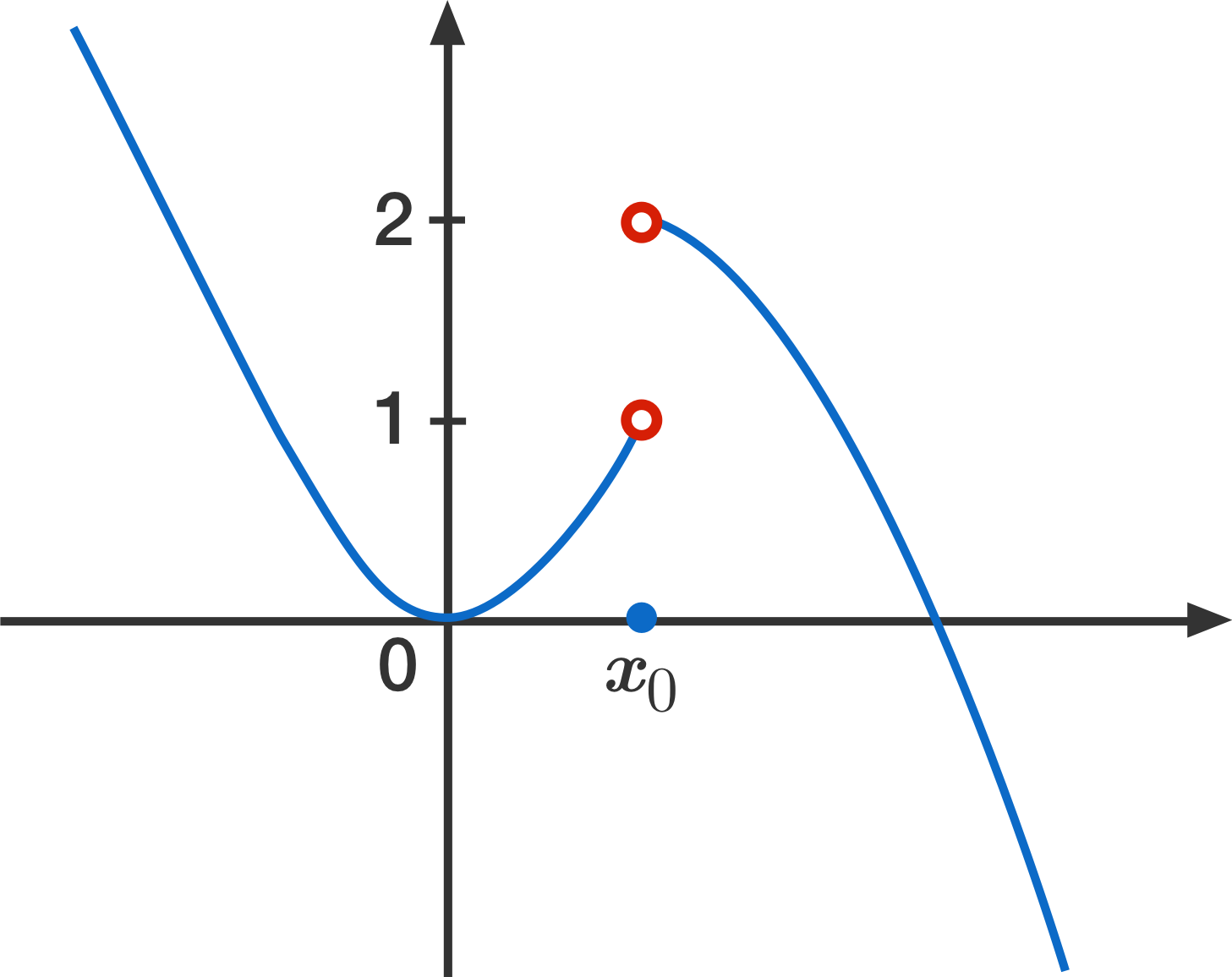



When Does A Limit Exist Brilliant Math Science Wiki
See the Indeterminate Forms wiki for further discussion Let m m m and n n n be positive integers FindIf lim ( ) x a f x l → = and lim ( ) x a g x m → =, then lim ( ) ( ) x a f x g x l m → ± = ± lim ( ) ( ) x a f x g x l m → ⋅ = ⋅ ( ) lim x a ( ) f x l → g x m = where m ≠ 0 lim ( ) x a c f x c l → ⋅ = ⋅ 1 1 lim x a→ f x l( ) = where l ≠ 0 Formulas 1 lim 1 n x e →∞ n = ( ) 1 lim 1 n x n e →∞ = 0 sin lim 1 x x → x = 0 tan lim 1 x x → x = 0 cos 1 lim 0 x x → x −Course Title MA 1023;




Subtraction Rule Of Limits
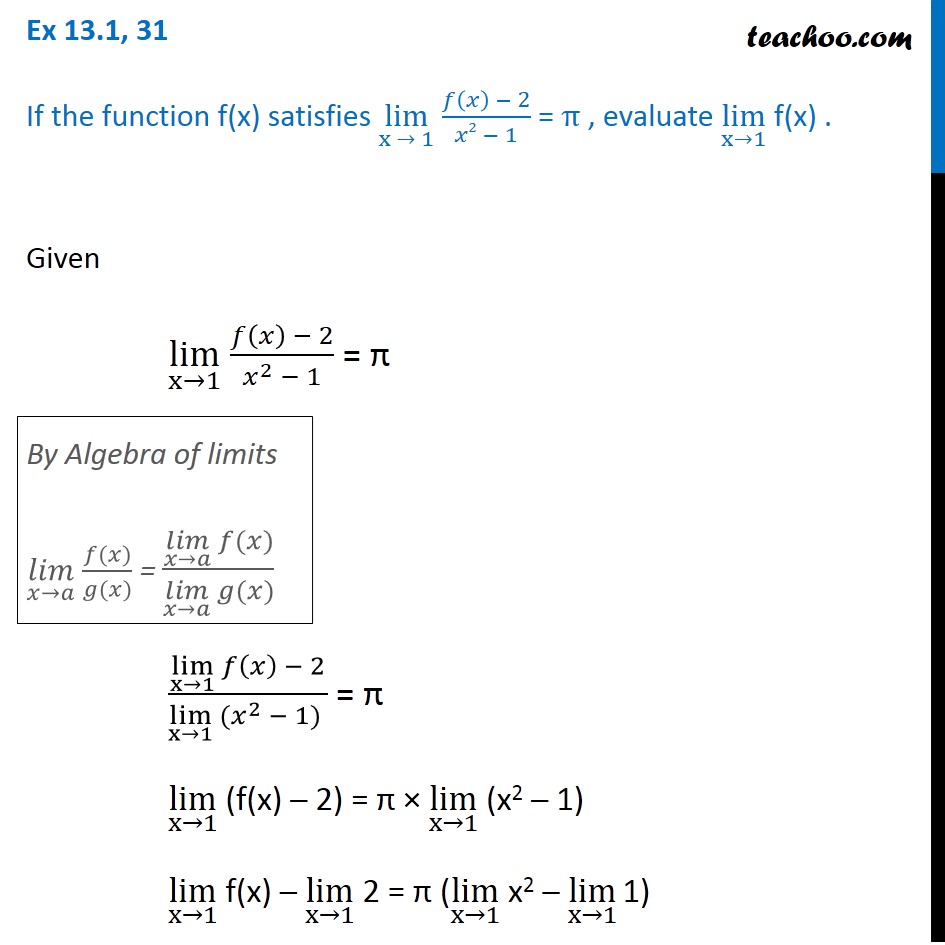



Ex 13 1 31 If Function F X Lim X 1 F X 2 X2 1 Pi



0 件のコメント:
コメントを投稿